This page contains automatically translated content.
Origami and other forms of mathematics
Math means above all: calculating? For Torsten Mütze, Professor of Discrete Mathematics, this is one of the misconceptions that A-level students come to university with. "Studying mathematics means above all reasoning and understanding structures." Two illustrative aids can convey this insight to first-year students in an easily digestible way: Origami and brain games. Mütze likes to use them.
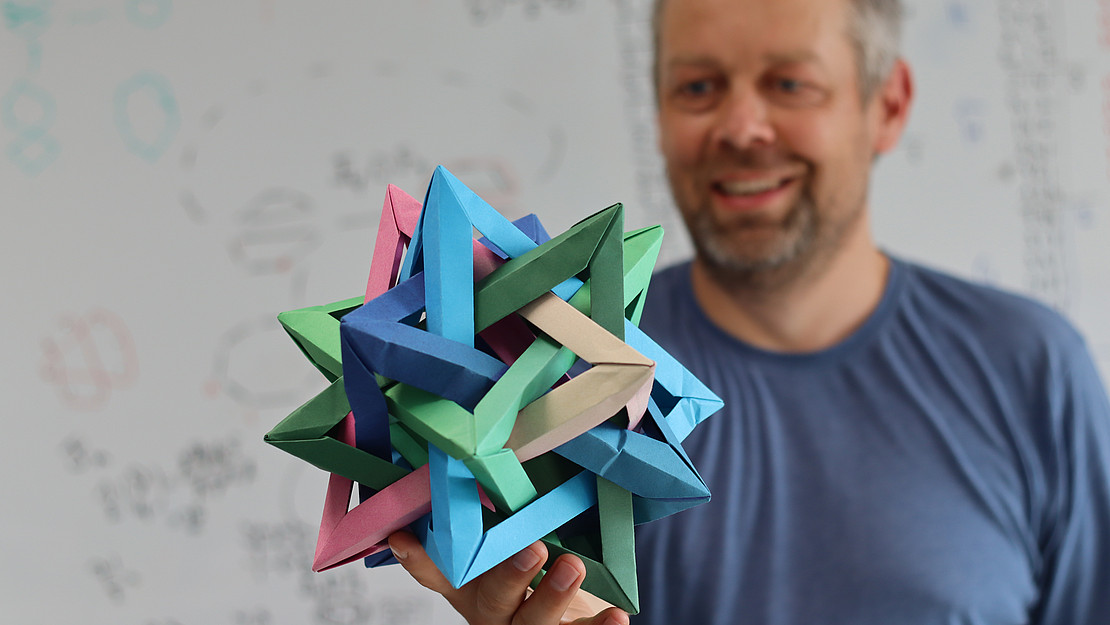 Image: Sebastian Mense
Image: Sebastian MenseTo put it casually, discrete mathematics deals with what can be expressed in ones and zeros - in contrast to continuous mathematics, which, to give a simple example, is used to specify temperatures: Here there is an infinite space of values between 14 and 15 degrees Celsius. If you think of computer science when you think of ones and zeros, you're not wrong. "The disciplines are closely interwoven," says Mütze. He studied computer science himself. At some universities, discrete mathematics is assigned to computer science.
Not so in Kassel. Mütze has been a professor at the Faculty of Mathematics and Natural Sciences in Oberzwehren since March. The German Research Foundation (DFG) is funding him with a so-called Heisenberg Professorship for outstanding young researchers. Before coming to Kassel, Mütze was an assistant professor at the University of Warwick in England.
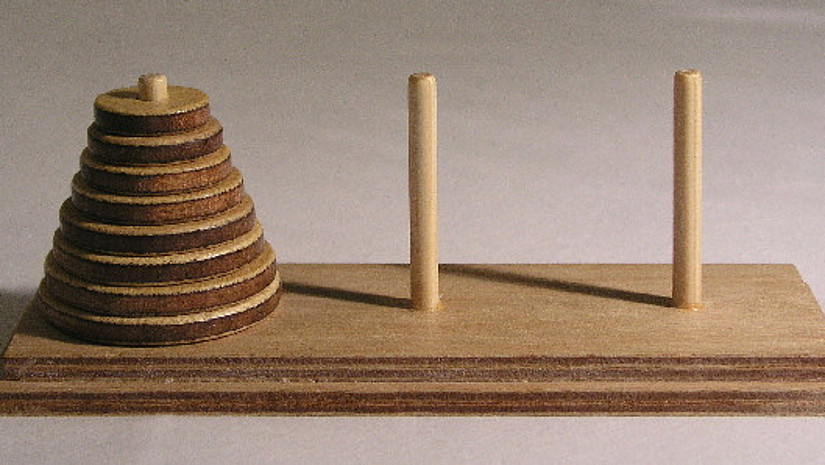
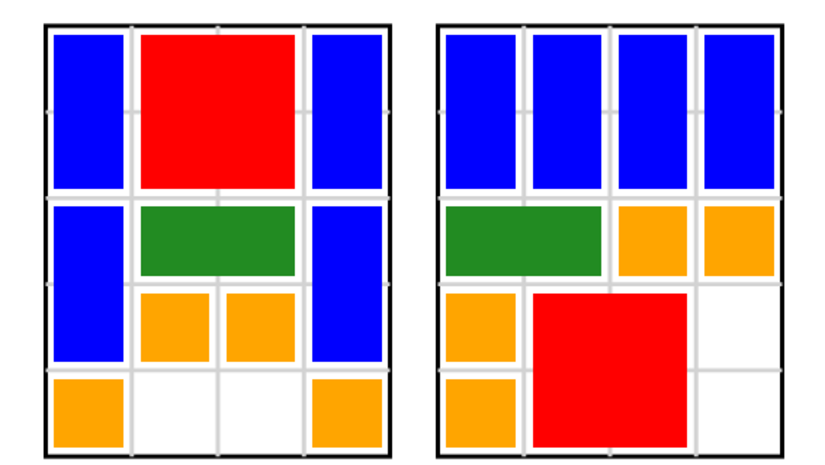
He combines a private curiosity with a professional interest in brain teasers. The best-known puzzle, Rubik's Rubik's Cube, could be found in every school playground in the 1980s. The interesting thing from the perspective of discrete mathematics is that every possible position of the Rubik's cube can be thought of as a node in a network. And the path to any other state - for example the goal: all colors are sorted - can be described as a graph. Anyone who understands graphs can also set up algorithms and is close to finding the solution for the Rubik's Cube. Incidentally, as mathematicians have discovered with the help of computers, it takes a maximum of 20 moves from each initial state of the cube to the ideal state sorted by color. Two other puzzle games that can be described using graphs are "Klotski" and "The Towers of Hanoi" (see below).
Mütze brings these games as well as the artistic origami objects into the lecture hall from time to time to illustrate facts. "In origami, there is a theorem that any figure with straight edges can be folded without ever using scissors," he explains. Even if you have to neglect the fact that in real life the paper will eventually become too thick - something like this can also be described wonderfully as a graph. Along the way, the students learn about "Platonic solids" (symmetrical 3-dimensional polyhedra) and other figures. Mütze: "Many of the students want to become teachers. This also gives them inspiration for their future teaching."
This article appeared in the university magazine publik 2024/3. Text and photo: Sebastian Mense